There are many difficult piano pieces. We present five pieces that are so demanding that they were considered unplayable at times. Even today, they still demand skills from pianists that cannot be acquired through practice alone.

Founder and Piano teacher
Intervals are the distances between any two tones. They form one of the foundations of musical theory and are therefore essential for many other areas of music, such as the theory of triads, tetrachords, chromatic steps, and improvisation.
Every interval has its own tonal character. Sooner or later, you will have to start learning how to identify each interval just by ear.
The theoretical principles are described in the following, to help you identify different intervals on paper.
Table of contents
Imagine sitting at the piano, your fingers gliding effortlessly across the keys as you play melodies that move your heart. With music2me, you can learn piano at your own pace – step by step with a system that truly helps you progress, whether you’re a beginner or already advanced.
Over 400 video lessons & downloadable sheet music
Interactive tools like Skill Check & smart practice mode
Weekly live classes & personal teacher support
Exclusive Discord community for motivation & exchange
In order to identify intervals, it’s helpful to know the chromatic scale.
Quarter tones and eighth-tones are often used in other cultures, but semitones are the smallest units in our Western music. On the piano, a semitone is the distance between two directly adjacent keys (e.g. C to C sharp, F to E).
The chromatic scale differs from the major scale in that it only exists of semitones. In other words, the scale contains all notes on the keyboard. The scale always moves to the next adjacent key.
The chromatic scale from Ab’ to Ab’’ is shown in the following illustration:
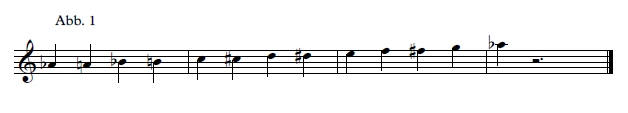
Let’s start with a large interval: the octave.
An octave is an interval in which the second note is the same note as the first one, but it’s the next lower or higher one.
The octave is made up of 12 semitones.
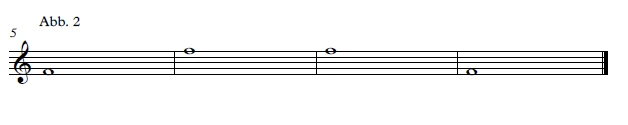
The illustration shows one octave step upwards, from F’ to F’’, and then again one octave down back to F’.

The illustration shows a major scale from F’ to B’’.
A major scale contains all diatonic intervals from the root (in this case, this is the F note, which is why this is called the F-major scale).
From the root (F’) to
F’, the interval is called the (perfect) prime (the interval between two equal notes)
G’, the interval is called the (major) second
A’, the interval is called the (major) third
B’, the interval is called the (perfect) fourth
C’’, the interval is called the (perfect) fifth
D’’, the interval is called the (major) sixth
E’’, the interval is called the (major) seventh
F’’, the interval is called the (perfect) octave
G’’, the interval is called the (major) ninth
A’’, the interval is called the (major) tenth (equal to a major third plus an octave)
B’’, the interval is called the (perfect) eleventh (equal to a perfect fourth plus an octave)
An altered interval is given by changing a diatonic interval by a semitone. In principle, there are five attributes given to interval names: diminished, minor, major, perfect, augmented.
Moving a major second/third/sixth/seventh down one semitone results in a minor second/third/sixth/seventh.
Moving a major second/third/sixth/seventh up one semitone results in an augmented second/third/sixth/seventh.
Moving a minor second/third/sixth/seventh down one semitone results in a diminished second/third/sixth/seventh.
There is no such thing as a perfect second/third/sixth/seventh. There are only perfect primes/fourths/fifths/octaves.
Moving a perfect prime/fourth/fifth/octave down one semitone results in a diminished prime/fourth/fifth/octave.
Moving a perfect prime/fourth/fifth/octave up one semitone results in an augmented prime/fourth/fifth/octave.
There is no such thing as a perfect prime/fourth/fifth/octave.
Of course, this all looks very complicated at first sight. There is, however, another way to determine intervals: using semitone steps.
The distance between C’ and C sharp is a semitone step. You start at C and “wander” across the board one key (or one step). This makes C’ and D’ two semitones apart. This way, a certain number of semitone steps can be assigned to each interval:
0 Semitone steps (STS) | Prime |
1 STS | Minor second/augmented prime |
2 STS | Major second/diminished third |
3 STS | Minor third/augmented |
4 STS | Major third/diminished fourth |
5 STS | Perfect fourth/augmented third |
6 STS | Augmented fourth/diminished fifth |
7 STS | Perfect fifth/diminished sixth |
8 STS | Minor sixth/augmented fifth |
9 STS | Major sixth/diminished seventh |
10 STS | Minor seventh/augmented sixth |
11 STS | Major seventh/diminished octave |
12 STS | Perfect octave/augmented seventh |
13 STS | Minor ninth/augmented octave (equals minor second plus an octave) |
14 STS | Major ninth/diminished tenth (major second plus an octave) |
15 STS | Minor tenth/augmented ninth (minor third plus an octave) |
16 STS | Major tenth/diminished eleventh (major third plus an octave) |
17 STS | Perfect eleventh/augmented tenth (perfect fourth plus an octave) |
18 STS | Augmented eleventh/diminished twelfth (augmented fourth plus an octave) |
You can use this table to work out how many semitone steps are in an interval.
Now, you might be wondering why there is more than one description in the right column of the table? Which description is the right one?
We’ll now discuss these enharmonic changes. An enharmonic change takes place whenever two notes with different names fall on the same key of the keyboard, and sound the same as well.
The reason why there are several descriptions for the same number of semitone steps is because of the altered intervals.
The major second (D) and major third (E) are two semitone steps apart. On the piano keyboard, you can see that there is another key between the two notes. Nevertheless, the major second can be altered one semitone upward (to get an augmented second) and the major third can be altered one semitone down (for a minor third). As a result, both descriptions apply to the key between them.
The notation indicates which description applies here:

On the left side of the illustration, there is an augmented second; on the right side is a minor third.
To the left, the diatonic interval “major second” (C to D) is altered one semitone up by the # sign. This results in an augmented second (C to D sharp).
To the right, the diatonic interval “major third” (C to E) is altered one semitone down by the b sign. This results in a minor third (C to E flat).
This principle, of both tones looking the same but appearing differently on the notation, is called an enharmonic change.
Try to recognise the intervals in the bars of the following illustration.
The solutions (intervals) of the 12 bars (from left to right) are given beneath the illustration.
(Don’t cheat!)

Solutions:
Perfect fifth
Major second
Minor sixth
Major third
Minor second
Diminished fifth
Minor seventh
Perfect fifth
Major ninth
Minor tenth (equals minor third plus an octave)
Augmented eleventh (equals augmented fourth plus an octave)
Perfect prime
There are many difficult piano pieces. We present five pieces that are so demanding that they were considered unplayable at times. Even today, they still demand skills from pianists that cannot be acquired through practice alone.
Is it even possible learning to play the piano as an adult? Of course it is! Discover how to play piano at any age.
Vivaldis Four Seasons is probably his most famous piece. We provide you its genesis and Vivaldis history.